A light particle moving horizontally with a s | vedclass

Name: PDF Generator App | JEE NEET Paper Generator | Vedclass
Brand: Vedclass
Rating: 5 (35 reviews)

Question

Conservation of momentum,

$m_{1} v_{1}+m_{2} v_{2}=m_{1} v_{1}^{\prime}+m_{2} v_{2}^{\prime}$

$\Rightarrow m_{1}\left(v_{1}-v_{1}^{\prime}\right

Vedclass · Accepted Answer

Move at $8\  m/s$ in its original direction

Vedclass · Answer

Conservation of momentum,

$m_{1} v_{1}+m_{2} v_{2}=m_{1} v_{1}^{\prime}+m_{2} v_{2}^{\prime}$

$\Rightarrow m_{1}\left(v_{1}-v_{1}^{\prime}\right)=m_{2}\left(v_{2}-v_{2}^{\prime}\right)&hellip;.(1)$

Conservation of kinetic energy requires,

$\frac{1}{2}\left(m_{1} v_{1}^{2}+m_{2} v_{2}^{2}\right)=\frac{1}{2}\left(m_{1} v_{1}^{\prime 2}+m_{2} v_{2}^{\prime 2}\right)$

$\Rightarrow m_{1}\left(v_{1}^{2}-v_{1}^{\prime 2}\right)=m_{2}\left(v_{2}^{2}-v_{2}^{\prime 2}\right)$

$\Rightarrow m_{1}\left(v_{1}-v_{1}^{\prime}\right)\left(v_{1}+v_{1}^{\prime}\right)=m_{2}\left(v_{2}-v_{2}^{\prime}\right)\left(v_{2}+v_{2}^{\prime}\right)&hellip;&hellip;(2)$

dividing $(2)$ and $(1)$

$v_{1}+v_{1}^{\prime}=v_{2}+v_{2}^{\prime}$

$12+v_{1}^{\prime}=10+10$

$v_{1}^{\prime}=8 m / s$

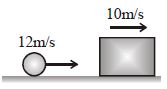
A light particle moving horizontally with a speed of $12\ m/s$ strikes a very heavy block moving in the same direction at $10\ m/s$. The collision is one-dimensional and elastic. After the collision, the particle will

Similar Questions

$Assertion$ : If collision occurs between two elastic bodies their kinetic energy decreases during the time of collision.

$Reason$ : During collision intermolecular space decreases and hence elastic potential energy increases.

Body of mass $M$ is much heavier than the other body of mass $m$. The heavier body with speed $v$ collides with the lighter body which was at rest initially elastically. The speed of lighter body after collision is

A bullet when fired at a target with a velocity of $100\,\,m/sec$ penetrates one metre into it. If the bullet is fired at a similar target with a thickness $0.5\,\,metre,$ then it will emerge from it with a velocity of

Two equal masses ${m_1}$ and ${m_2}$ moving along the same straight line with velocities $+ 3 \,m/s$ and $-5\, m/s$ respectively collide elastically. Their velocities after the collision will be respectively

The quantities remaining constant in a collision are