A mass of $4\; kg$ rests on a horizontal plane. The plane is gradually inclined until at an angle $\theta= 15^o$ with the horizontal, the mass just begins to slide. What is the coefficient of static friction between the block and the surface ?
A mass of $4\; kg$ rests on a horizontal plane. The plane is gradually inclined until at an angle $\theta= 15^o$ with the horizontal, the mass just begins to slide. What is the coefficient of static friction between the block and the surface ?
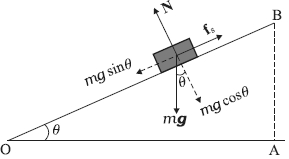
Answer The forces acting on a block of mass $m$ at rest on an inclined plane are $(i)$ the weight mg acting vertically downwards $(ii)$ the normal force $N$ of the plane on the block, and $(iii)$ the static frictional force $f_{ s }$ opposing the impending motion. In equilibrium, the resultant of these forces must be zero. Resolving the weight $m g$ along the two directions shown, we have
$m g \sin \theta=f_{s}, \quad m g \cos \theta=N$
As $\theta$ increases, the self-adjusting frictional force
$f_{ s }$ increases until at $\theta=\theta_{\max ^{\prime}} f_{ s }$ achieves its
maximum value, $\left(f_{s}\right)_{\max }=\mu_{s} N$
Therefore.
$\tan \theta_{\max }=\mu_{s}$ or $\theta_{\max }=\tan ^{-1} \mu_{s}$
When $\theta$ becomes Just a little more than $\theta_{\max }$. there is a small net force on the block and it begins to slide. Note that $\theta_{\max }$ depends only on
$\mu_{ s }$ and $1 s$ independent of the mass of the block.
For $\quad \theta_{\max }=15^{\circ}$
$\mu_{s}=\tan 15^{\circ}$
$=0.27$
Similar Questions
A body takes $1\frac{1}{3}$ times as much time to slide down a rough identical but smooth inclined plane. If the angle of inclined plane is $45^o$, the coefficient of friction is
A body takes $1\frac{1}{3}$ times as much time to slide down a rough identical but smooth inclined plane. If the angle of inclined plane is $45^o$, the coefficient of friction is
A box of mass $m\, kg$ is placed on the rear side of an open truck accelerating at $4\, m/s^2$. The coefficient of friction between the box and the surface below it is $0.4$. The net acceleration of the box with respect to the truck is zero. The value of $m$ is :- $[g = 10\,m/s^2]$
A box of mass $m\, kg$ is placed on the rear side of an open truck accelerating at $4\, m/s^2$. The coefficient of friction between the box and the surface below it is $0.4$. The net acceleration of the box with respect to the truck is zero. The value of $m$ is :- $[g = 10\,m/s^2]$
The rear side of a truck is open and a box of $40 \;kg$ mass is placed $5 \,m$ away from the open end as shown in Figure. The coeffictent of friction between the box and the surface below it is $0.15 .$ On a stratght road, the truck starts from rest and accelerates with $2\; m s ^{-2} .$ At what distance from the starting point does the box fall off the truck? (Ignore the size of the box).
The rear side of a truck is open and a box of $40 \;kg$ mass is placed $5 \,m$ away from the open end as shown in Figure. The coeffictent of friction between the box and the surface below it is $0.15 .$ On a stratght road, the truck starts from rest and accelerates with $2\; m s ^{-2} .$ At what distance from the starting point does the box fall off the truck? (Ignore the size of the box).
The limiting friction between two bodies in contact is independent of
The limiting friction between two bodies in contact is independent of
When a body slides down from rest along a smooth inclined plane making an angle of $45^o$ with the horizontal, it takes time $T$. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it is seen to take time $pT$, where $p$ is some number greater than $1$. Calculate the coefficient of friction between the body and the rough plane.
When a body slides down from rest along a smooth inclined plane making an angle of $45^o$ with the horizontal, it takes time $T$. When the same body slides down from rest along a rough inclined plane making the same angle and through the same distance, it is seen to take time $pT$, where $p$ is some number greater than $1$. Calculate the coefficient of friction between the body and the rough plane.