If two glass plates have water between them and are separated by very small distance ( see figure), it is very difficult to pull them apart. It is because the water in between forms cylindrical surface on the side that gives rise to lower pressure in the water in comparison to atmosphere. If the radius of the cylindrical surface is $R$ and surface tension of water is $T$ then the pressure in water between the plates is lower by
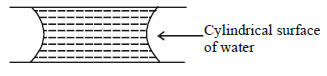
If two glass plates have water between them and are separated by very small distance ( see figure), it is very difficult to pull them apart. It is because the water in between forms cylindrical surface on the side that gives rise to lower pressure in the water in comparison to atmosphere. If the radius of the cylindrical surface is $R$ and surface tension of water is $T$ then the pressure in water between the plates is lower by

- [JEE MAIN 2015]
- A
$\frac {T}{R}$
- B
$\frac {4T}{R}$
- C
$\frac {T}{4R}$
- D
$\frac {2T}{R}$
Similar Questions
Fill in the Blank :
$(i)$ Bubble in water have .......... free surface.
$(ii)$ Bubble in air have .......... free surface.
$(iii)$ Rain drop have .......... free surface.
Fill in the Blank :
$(i)$ Bubble in water have .......... free surface.
$(ii)$ Bubble in air have .......... free surface.
$(iii)$ Rain drop have .......... free surface.
The pressure inside a small air bubble of radius $0.1\, mm$ situated just below the surface of water will be equal to [Take surface tension of water $70 \times {10^{ - 3}}N{m^{ - 1}}$ and atmospheric pressure = $1.013 \times {10^5}N{m^{ - 2}}$]
The pressure inside a small air bubble of radius $0.1\, mm$ situated just below the surface of water will be equal to [Take surface tension of water $70 \times {10^{ - 3}}N{m^{ - 1}}$ and atmospheric pressure = $1.013 \times {10^5}N{m^{ - 2}}$]
When two soap bubbles of radii $a$ and $b ( b > a )$ coalesce, the radius of curvature of common surface is
When two soap bubbles of radii $a$ and $b ( b > a )$ coalesce, the radius of curvature of common surface is
- [JEE MAIN 2021]
The excess of pressure inside a soap bubble is twice the excess pressure inside a second soap bubble. The volume of the first bubble is $n$ times the volume of the second where $n$ is
The excess of pressure inside a soap bubble is twice the excess pressure inside a second soap bubble. The volume of the first bubble is $n$ times the volume of the second where $n$ is
Write the equation of excess pressure for liquid drop.
Write the equation of excess pressure for liquid drop.
